Codeforces Round 900 (Div. 3)(A-F)
比赛链接? :
Dashboard - Codeforces Round 900 (Div. 3) - Codeforces
A. How Much Does Daytona Cost?
题面 :?

思路 :
在序列中只要找到k,就返回true ;
代码 :?
#include<bits/stdc++.h>
#define IOS ios::sync_with_stdio(0);cin.tie(0);cout.tie(0);
#define endl '\n'
using namespace std;
typedef long long LL;
const int N = 2e5+10;
inline void solve(){
int n , k ; cin >> n >> k ;
bool tag = false;
for(int i=0;i<n;i++){
int x ; cin >> x ;
if(x==k) tag = true;
}
if(tag) cout << "Yes" << endl;
else cout << "No" << endl;
return ;
}
int main()
{
IOS
int _ = 1;
cin >> _;
while(_ --) solve();
return 0;
}B. Aleksa and Stack
题面 :?

思路 :
在这道题中,只要满足任意两个相邻数的和,不能够不是3的倍数,且数组单调递增,那么便可以构造出这样一个序列,每两个相邻数中第一个数 mod 3 = 0,另一个数mod 3 = 1 ,然后递增的话,就可以使a1 = 3 *1 , a2= a1 + 1,a3 = 3 * 2,a4 = a3 + 1,即可满足题目条件;
代码
#include<bits/stdc++.h>
#define IOS ios::sync_with_stdio(0);cin.tie(0);cout.tie(0);
#define endl '\n'
using namespace std;
typedef long long LL;
int gcd(int a,int b){ return b==0 ? a : gcd(b,a%b); }
int lcm(int a,int b){ if(a==0||b==0) return 0; return (a*b)/gcd(a,b); }
bool is_prime(int x){if(x<2) return false;
for(int i=2;i<=x/i;i++) if(x%i==0) return false; return true;}
//numbers.erase(std::unique(numbers.begin(), numbers.end()), numbers.end()); // 去重操作
const int N = 2e5+10;
// 任意两个数的和不是3的倍数
// 0, 1, 2
// 3 , 4 , 6, 7
inline void solve(){
int n ; cin >> n ;
int x = 3;
while(n>1){
cout << x << " " << x + 1 << " ";
x += 3;
n -= 2;
}
if(n) cout << x ;
cout << endl ;
return ;
}
int main()
{
IOS
int _ = 1;
cin >> _;
while(_ --) solve();
return 0;
}?C. Vasilije in Cacak
题面 :?
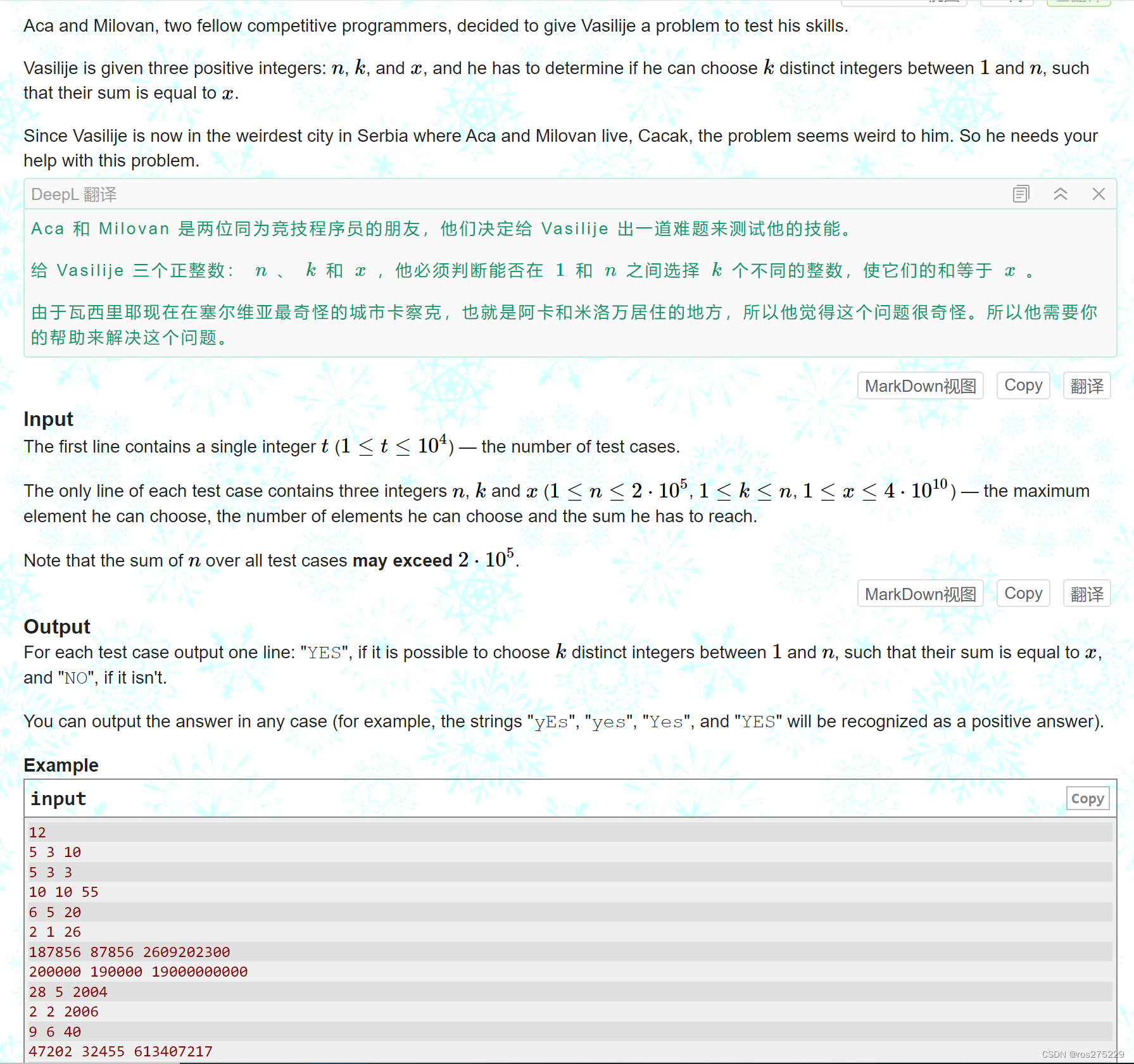
思路 :?
对于从??[1 ,n ]? 中 选k个数 的 和为x;
假如 n = 2 (并且假设k = 2,下面一样):
?????????1,2 --> 1, 2, 3
n = 3 :
????????1, 2 ,3 : 1,2,3,4,5,6 选两个 : 3-5?
n = 4 :
????????1,2,3,4 : 3-7 : ?
那么我们就可以发现一个规律 : 在[1,n]中取k个数和的范围是[最小的k个数相加,最大的k个数相加];
为了计算的方便,我们可以使用等差数列求和 : s = n*a1+(n-1)*n*d/2? ;
代码 :?
#include<bits/stdc++.h>
#define IOS ios::sync_with_stdio(0);cin.tie(0);cout.tie(0);
#define endl '\n'
using namespace std;
typedef long long LL;
LL n , k , x ;
// [1,n] 中 选k个数 的 和为x;
// 1,2 --> 1, 2, 3
//1, 2 ,3 : 1,2,3,4,5,6 选两个 : 3-5
// 1,2,3,4 : 3-7 :
// 等差数列求和 : s = n*a1+(n-1)*n*d/2
inline void solve(){
cin >> n >> k >> x;
LL l = (1+k)*k/2 , r = (n+n-k+1)*k/2;
if(x>=l && x<=r) cout << "Yes" << endl;
else cout << "No" << endl;
return ;
}
int main()
{
IOS
int _ = 1;
cin >> _;
while(_ --) solve();
return 0;
}D. Reverse Madness
题面

思路 :?
l和r都是单调递增的,且对于每一段区间都是不相交的,所有可以很快找到x对应的li和ri来满足li<=x && x<=ri ;
找到之后就是进行区间的反转,对于每个区间假设li = 2,ri =6,如果x = 4,则a=b=4,如果x=3,则a=3,b=5;那么说明是关于终点对称的,那么就可以使用前缀和算法,来对每个点进行反转次数的统计,如果统计次数为偶那就不用反转了,为奇,则要反转,具体请看代码;
代码
#include<bits/stdc++.h>
#define IOS ios::sync_with_stdio(0);cin.tie(0);cout.tie(0);
#define endl '\n'
using namespace std;
typedef long long LL;
const int N = 2e5+10;
inline void solve(){
int n,k;cin >> n >> k ;
string s ; cin >> s ;
s = ' ' + s ;
vector<int> l(k+1) , r (k+1) ;
for(int i=1;i<=k;i++) cin >> l[i] ;
for(int i=1;i<=k;i++) cin >> r[i] ;
int q ; cin >> q ;
vector<int> x(q+1) ;
for(int i=1;i<=q;i++) cin >> x[i] ;
vector<pair<int,int>> sum(n+3) ;
for(int i=1;i<=q;i++){
int pos = lower_bound(r.begin()+1,r.end(),x[i]) - r.begin();
int a = min(x[i] , r[pos]+l[pos]-x[i]);
int b = max(x[i],r[pos]+l[pos]-x[i]);
sum[a].first++ ; sum[b+1].first--;
sum[a].second = b ; sum[b].second = a ;
}
for(int i=1;i<n;i++){
sum[i].first += sum[i-1].first ;
}
int start = -1 , end = -1 ;
for(int i=1;i<=n;i++){
if(sum[i].first % 2 == 1){
start = i ;
int end = sum[i].second ;
while(start <= end){
while(start<=end && sum[start].first % 2 == 1){
swap(s[start],s[end]);
start ++;
end--;
}
while(start <= end && sum[start].first % 2 == 0){
start ++;
end -- ;
}
}
i = sum[i].second ;
}
}
for(int i=1;i<=n;i++) cout << s[i] ;
cout << endl;
}
int main()
{
IOS
int _ = 1;
cin >> _;
while(_ --) solve();
return 0;
}E. Iva & Pav
题面 :

思路 :?
// f(l,r)=al & al+1 &…& ar
// 给定一个 l , k ,求最大的r,满足f(l , r) >= k ;
// 定l,则f随着r单调递减?
// & : 按位与?
按位与的特点是,对于某一位,[l,r]上的所有数的该位上为1,结果才为1,那么我们可以采用前缀和的思想,用bit[i][j]来存[1,i]上第j位上为1的个数,具体实现请看代码,再获得bit数组之后,因为在l确定之后,f(l,r)随着r的增大具有单调递减的性质,所有可以使用二分来进行操作;
具体实现请看代码 ;
代码 :?
// f(l,r)=al & al+1 &…& ar
// 给定一个 l , k ,求最大的r,满足f(l , r) >= k ;
// 定l,则f随着r单调递减
// & : 按位与
#include<bits/stdc++.h>
#define IOS ios::sync_with_stdio(0);cin.tie(0);cout.tie(0);
#define endl '\n'
using namespace std;
typedef long long LL;
const int N = 2e5 + 10 ;
inline void solve(){
int n ; cin >> n ;
vector<array<int,32>> bit(n+1);
for(int i=1;i<=n;i++){
int x ; cin >> x ;
for(int j = 0;j<32;j++){
bit[i][j] = 0 ;
bit[i][j] += x % 2 ;
x /= 2 ;
bit[i][j] += bit[i-1][j] ; // 进行前缀和操作,统计i前面j位上为1的个数
}
}
auto check = [&](int l,int r ,int c){
vector<int> b(32) ;
for(int i=0;i<32;i++){
b[i] = bit[r][i] - bit[l-1][i] ;
}
int ans = 0 ;
for(int i=0;i<32;i++){
if(b[i]==r-l+1) ans += pow(2,i);
}
return ans >= c ;
};
int q ; cin >> q ;
while(q--){
int l , k ; cin >> l >> k ;
int L = l, R = n;
int ans = -1 ;
while(L<=R){
int mid = (L+R)>>1 ;
if(check(l,mid,k)){
ans = mid ;
L = mid + 1;
}else{
R = mid - 1 ;
}
}
cout << ans << " " ;
}
cout << endl ;
}
int main()
{
IOS
int _ = 1;
cin >> _;
while(_ --) solve();
return 0;
}F. Vasilije Loves Number Theory
题面 :?

思路 :?
- ?1 : n *= x,然后问是否存在一个a使得gcd(n,a)=1并且n*a的约数个数等于n,
- gcd(n,a)=1 --> n,a互质
- 由于n,a互质,那么 d(n)*d(a)=d(n*a),那么就是要d(a) = n / d(n),所以n % d(n)一定要等于零?
然后就可以通过唯一分解定理来解;
具体实现请看代码 :
代码
#include<bits/stdc++.h>
#define IOS ios::sync_with_stdio(0);cin.tie(0);cout.tie(0);
#define endl '\n'
using namespace std;
typedef long long LL;
const int N = 2e5+10;
// 1 : n *= x,然后问是否存在一个a使得gcd(n,a)=1并且n*a的约数个数等于n,
// gcd(n,a)=1 --> n,a互质
// --> d(n)*d(a)=d(n*a),那么就是要d(a) = n / d(n),所以n % d(n)一定要等于零
inline void solve(){
int n,q;cin>>n>>q;
int cnt = 1 ; // 记录因数的数量
map<int,int> doc ;
// 质因数分解 :
// num = b1^c1 + b2 ^c2 + .... + bn^cn ;
for(int i=2;i*i<=n;i++){
// i相当于上面的b,c相当于上面的c ;
if(n%i==0){
int c = 0 ;
while(n%i==0) n/=i,c++;
doc[i] = c ;
cnt *= (c+1);
}
}
if(n>1) doc[n] = 1 , cnt *= 2 ; // 最后剩下的n本身也是一个质因数
int now = cnt;auto doc2 = doc ;
while(q--){
int op ; cin >> op ;
if(op == 2){
now = cnt ;
doc2 = doc ;
}else{
int x ; cin >> x ;
for(int i=2;i*i<=x;i++){
if(x%i==0){
int c = 0 ;
while(x%i==0) x/=i,c++;
now /= (doc2[i]+1);
doc2[i] += c;
now *= (doc2[i]+1);
}
}
if(x>1){
now /= (doc2[x]+1);
doc2[x]+=1;
now *= (doc2[x]+1);
}
int t = now ;
for(auto it:doc2){
int x = it.first ;
int y = it.second ;
while(y>0 && t % x== 0){
t /= x ;
y -- ;
}
}
cout << (t==1?"YES":"NO")<<endl;
}
}
}
int main()
{
IOS
int _ = 1;
cin >> _;
while(_ --) solve();
return 0;
}本文来自互联网用户投稿,该文观点仅代表作者本人,不代表本站立场。本站仅提供信息存储空间服务,不拥有所有权,不承担相关法律责任。 如若内容造成侵权/违法违规/事实不符,请联系我的编程经验分享网邮箱:veading@qq.com进行投诉反馈,一经查实,立即删除!