leetcode - 1901. Find a Peak Element II

Description

A peak element in a 2D grid is an element that is strictly greater than all of its adjacent neighbors to the left, right, top, and bottom.

Given a 0-indexed m x n matrix mat where no two adjacent cells are equal, find any peak element mat[i][j] and return the length 2 array [i,j].

You may assume that the entire matrix is surrounded by an outer perimeter with the value -1 in each cell.

You must write an algorithm that runs in O(m log(n)) or O(n log(m)) time.

Example 1:

Input: mat = [[1,4],[3,2]]
Output: [0,1]
Explanation: Both 3 and 4 are peak elements so [1,0] and [0,1] are both acceptable answers.

Example 2:

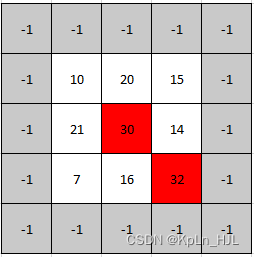
Input: mat = [[10,20,15],[21,30,14],[7,16,32]]
Output: [1,1]
Explanation: Both 30 and 32 are peak elements so [1,1] and [2,2] are both acceptable answers.

Constraints:

m == mat.length
n == mat[i].length
1 <= m, n <= 500
1 <= mat[i][j] <= 105
No two adjacent cells are equal.

Solution

$o(m?n)$ time compleixty

Find the maximum of each column or row, and find the peak element of those maximum elements.

Time complexity: $o(m?n)$ for finding the maximum elements, $n\log m)$ for finding the peak element.

$o(n\log m)$ time complexity

We don’t need to calculate the maximum of each row, instead we just need to know the maximum of midRow, and compare it with the adjacent rows. discard the smaller half.

By doing this, we could make sure we find the globally peak.

Time complexity: $o(n\log m)$
Space complexity: $o(1)$

Code

$o(m?n)$ time compleixty

class Solution:
    def findPeakGrid(self, mat: List[List[int]]) -> List[int]:
        m, n = len(mat), len(mat[0])
        candidates = []
        for i in range(m):
            cur_max = -1
            cur_max_index = -1
            for j in range(n):
                if mat[i][j] > cur_max:
                    cur_max = mat[i][j]
                    cur_max_index = j
            candidates.append((cur_max, cur_max_index))
        left, right = 0, len(candidates) - 1
        while left < right:
            mid = (left + right) >> 1
            if (mid - 1 < 0 or candidates[mid - 1][0] < candidates[mid][0]) and (mid + 1 >= len(candidates) or candidates[mid + 1][0] < candidates[mid][0]):
                return [mid, candidates[mid][1]]
            elif mid - 1 < 0 or candidates[mid - 1][0] < candidates[mid][0]:
                left = mid + 1
            elif mid + 1 >= len(candidates) or candidates[mid + 1][0] < candidates[mid][0]:
                right = mid
            else:
                left = mid + 1
        mid = (left + right) >> 1
        return [mid, candidates[mid][1]]

$o(n\log m)$ time complexity

class Solution:
    def findPeakGrid(self, mat: List[List[int]]) -> List[int]:
        m, n = len(mat), len(mat[0])
        left, right = 0, m - 1
        while left < right:
            mid = (left + right) >> 1
            # find maximum on midth row
            cur_max, cur_max_index = -1, -1
            for col in range(0, n):
                if mat[mid][col] > cur_max:
                    cur_max = mat[mid][col]
                    cur_max_index = col
            if (mid - 1 < 0 or mat[mid - 1][cur_max_index] < mat[mid][cur_max_index]) and (mid + 1 >= m or mat[mid + 1][cur_max_index] < mat[mid][cur_max_index]):
                return [mid, cur_max_index]
            elif mid - 1 < 0 or mat[mid - 1][cur_max_index] < mat[mid][cur_max_index]:
                left = mid + 1
            elif mid + 1 >= m or mat[mid + 1][cur_max_index] < mat[mid][cur_max_index]:
                right = mid
            else:
                left = mid + 1
        mid = (left + right) >> 1
        # find maximum on midth row
        cur_max, cur_max_index = -1, -1
        for col in range(0, n):
            if mat[mid][col] > cur_max:
                cur_max = mat[mid][col]
                cur_max_index = col
        return [(left + right) >> 1, cur_max_index]