回溯（dfs）题集（1）

在这里主要是记录我Leetcode刷题所写的代码

78子集

class Solution {  
    // 存储结果的列表，每个子列表代表一种子集  
    List<List<Integer>> ans = new ArrayList<>();  
    // 临时存储当前子集的列表  
    List<Integer> re = new ArrayList<>();  
  
    // 主方法，返回给定数组的所有子集  
    public List<List<Integer>> subsets(int[] nums) {  
        // 调用深度优先搜索方法  
        dfs(nums, 0);  
        // 返回所有子集  
        return ans;  
    }  
  
    // 深度优先搜索方法，使用回溯的思想来生成所有可能的子集  
    public void dfs(int[] nums, int start) {  
        // 将当前子集添加到结果列表中  
        ans.add(new ArrayList<>(re));  
        // 如果已经遍历完数组，则返回（相当于递归的结束条件）  
        if(start >= nums.length) return;  
        // 从当前位置开始遍历数组  
        for(int i = start; i < nums.length; i++) {   
            // 将当前元素添加到临时子集中  
            re.add(nums[i]);  
            // 继续递归，从下一个位置开始遍历  
            dfs(nums, i + 1);  
            // 回溯，移除临时子集中的最后一个元素，尝试其他可能的组合  
            re.removeLast();  
        }  
    }  
}

17 电话号码的字母组合

class Solution {  
    // 存储结果的列表，每个字符串代表一种组合  
    List<String> ans = new ArrayList<>();  
    // 输入的数字字符串长度  
    int n;  
    // 临时存储当前组合的字符串  
    StringBuffer sb = new StringBuffer();  
  
    // 主方法，返回给定数字字符串的所有字母组合  
    public List<String> letterCombinations(String digits) {  
        // 如果输入为空，则直接返回结果列表  
        if(digits.length() == 0)    return ans;  
        // 定义一个字符串数组，每个数字对应一个字母集合  
        String[] numstring = {"", "","abc","def","ghi","jkl","mno","pqrs","tuv","wxyz"};  
        // 调用深度优先搜索方法  
        dfs(0, numstring, digits);  
        // 返回所有字母组合  
        return ans;  
    }  
  
    // 深度优先搜索方法，使用回溯的思想来生成所有可能的字母组合  
    public void dfs(int idx, String[] numstring, String digits) {  
        // 如果当前组合的长度等于输入数字字符串的长度，则将组合添加到结果列表中  
        if(sb.length() == digits.length()) {  
            ans.add(new String(sb));  
            return;  
        }  
        // 获取当前数字对应的字母集合  
        String now = numstring[digits.charAt(idx) - '0'];  
        // 遍历当前字母集合中的每个字母，并递归调用dfs方法  
        for(int i = 0;i < now.length();i ++) {  
            sb.append(now.charAt(i));  // 将当前字母添加到临时组合中  
            dfs(idx + 1, numstring, digits);  // 递归调用，处理下一个数字字符  
            sb.deleteCharAt(sb.length() - 1);  // 回溯，移除临时组合中的最后一个字母，尝试其他可能的组合  
        }  
    }  
}

39组合总和

这是我一开始写的代码：

class Solution {
    List<List<Integer>> ans = new ArrayList<>();
    List<Integer> re = new ArrayList<>();
    boolean[] state;
    public List<List<Integer>> combinationSum(int[] candidates, int target) {
        Arrays.sort(candidates);
        state = new boolean[candidates.length + 1];
        dfs(candidates, target, 0);
        return ans;
    }
    public void dfs(int[] candidates, int target, int now) {
        if(now == target) {
            ans.add(new ArrayList<>(re));
            return;
        } else if(now > target) {
            return;
        }
        for(int i = 0;i < candidates.length;i ++) {
            if(!state[i]) {
                now += candidates[i];
                re.add(candidates[i]);
                // state[i] = true;
                dfs(candidates, target, now);
                now -= candidates[i];
                re.removeLast();
                // state[i] = false;
            }
        }
    }
} 

但是测试用例是这样的：

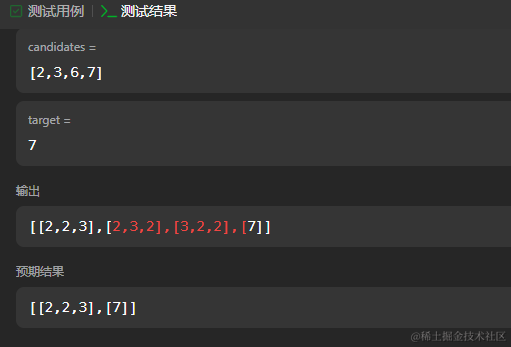
也就是说我实现了排列，但是没实现组合,看了题解，还是没从当前位置继续遍历的问题

class Solution {
    List<List<Integer>> ans = new ArrayList<>();
    List<Integer> re = new ArrayList<>();
    boolean[] state;
    public List<List<Integer>> combinationSum(int[] candidates, int target) {
        // Arrays.sort(candidates);
        state = new boolean[candidates.length + 1];
        dfs(candidates, target, 0, 0);
        return ans;
    }
    public void dfs(int[] candidates, int target, int now, int start) {
        if(now == target) {
            ans.add(new ArrayList<>(re));
            return;
        } else if(now > target) {
            return;
        }
        for(int i = start;i < candidates.length;i ++) {
            now += candidates[i];
            re.add(candidates[i]);
            dfs(candidates, target, now, i);
            now -= candidates[i];
            re.removeLast();
        
        }
    }
} 

22 括号生成

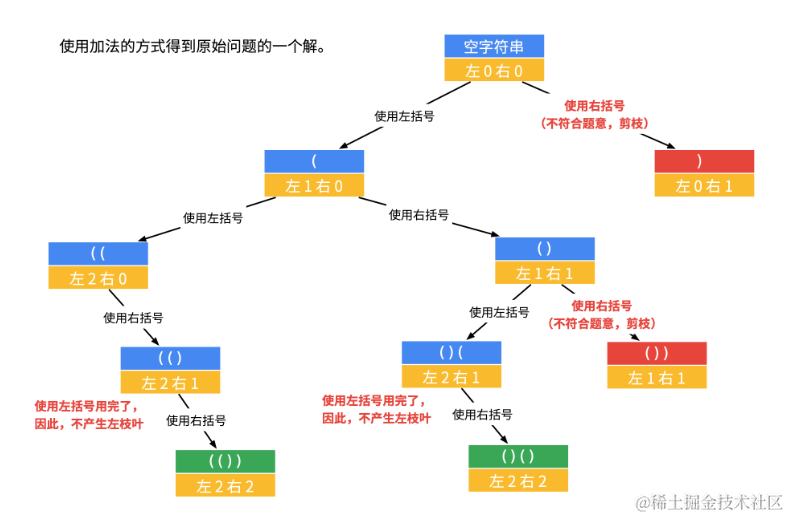
感谢题解里liweiwei1419的思路解答，我知道肯定用dfs进行搜索，可是不知道如何匹配，这个就教会我如何匹配了，因为知道用dfs其实就是套模板了，但是要知道如何去匹配这个。

class Solution {
    public List<String> generateParenthesis(int n) {
        List<String> ans = new ArrayList<>();
        if(n == 0)  return ans;
        dfs("", 0, 0, n, ans);
        return ans;
    }
    public void dfs(String s, int left, int right, int n,List<String> ans) {
        if(left == n && right == n) {
            ans.add(s);
            return;
        }
        // 不符合条件退出, 剪枝
        if(left < right) return;
        // 先左边
        if(left < n) {
            dfs(s + '(', left + 1, right, n, ans);
        }
        // sb.deleteCharAt(sb.length() - 1);
        if(right < n) {
            dfs(s + ')', left, right + 1, n, ans);
        }
    }
}

79 单词搜索

class Solution {
    String target;
    int m,n;
    public boolean exist(char[][] board, String word) {
        target = word;
        m = board.length;
        n = board[0].length;
        for(int i = 0;i < m;i ++) {
            for(int j = 0;j < n;j ++) {
                if(dfs(board, i, j, 0)) {
                    return true;
                }
            }
        }
        return false;
    }
    public boolean dfs(char[][] board, int x, int y, int idx) {
        if(x >= m || x < 0 || y >= n || y < 0 || board[x][y] != target.charAt(idx)) return false;
        if(idx == target.length() - 1) return true;
        // 利用回溯，所以要设置一个temp值
        char temp = board[x][y];
        board[x][y] = '.';
        // 遍历周围
        boolean b = (dfs(board, x, y + 1, idx + 1) || dfs(board, x, y - 1, idx + 1) || 
        dfs(board, x + 1, y, idx + 1) || dfs(board, x - 1, y, idx + 1));
        board[x][y] = temp;
        return b;
    }
}