AcWing 889. 满足条件的01序列(卡特兰数应用)
满足条件的01序列
假设长度为n个序列要求满足题意1的前缀0的个数不能超过1的个数
将问题抽象为从(0, 0)到(n, n)
向上走一个代表这一步对应序列中的值是1,向右走代表序列中的值是0
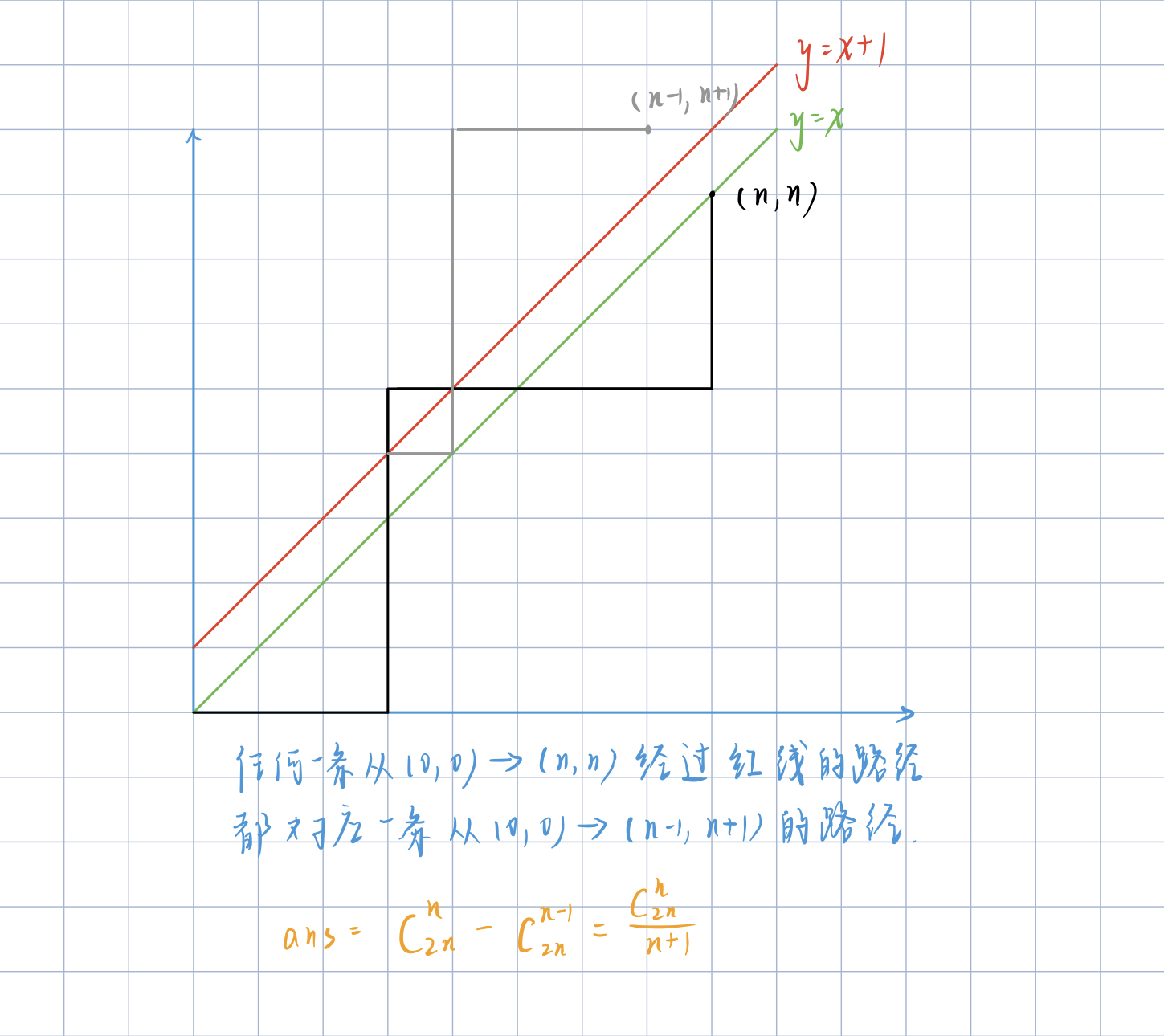
要想满足1的前缀0的数量大于1的数量就需要满足所有路过的途径在y = x这个函数个下面
但是如何表达呢?
我们采用所有到(n, n)的方案的集合减去越过y = x + 1这个直线的方案集合
因为越过y = x + 1 这个直线的方案集合可以表示为从(0, 0)到(n - 1, n + 1){(n, n)关于 y = x + 1对称的点}的方案集合
而这个答案
C
(
n
2
n
)
?
C
(
n
?
1
2
n
)
C\binom{n}{2n} - C\binom{n - 1}{2n}
C(2nn?)?C(2nn?1?)
=
2
n
!
n
!
?
n
!
?
2
n
!
(
n
+
1
)
!
(
n
?
1
)
!
= \frac{2n!}{n!*n!} - \frac{2n!}{(n + 1)!(n - 1)!}
=n!?n!2n!??(n+1)!(n?1)!2n!?
= 2 n ! ? ( n + 1 ) n ! ? ( n + 1 ) ! ? 2 n ! ? n n ! ? ( n + 1 ) ! = \frac{2n!*(n + 1)}{n! * (n + 1)!} - \frac{2n! * n}{n! * (n + 1)!} =n!?(n+1)!2n!?(n+1)??n!?(n+1)!2n!?n?
=
1
(
n
+
1
)
2
n
!
n
!
?
n
!
= \frac{1}{(n + 1)} \frac{2n!}{n!*n!}
=(n+1)1?n!?n!2n!?
称为卡特兰数
=
C
(
n
2
n
)
n
+
1
= \frac{C\binom{n}{2n}}{n + 1}
=n+1C(2nn?)?
2n是x的跨度和y的跨度的和
#include <iostream>
#include <cstring>
#include <algorithm>
using namespace std;
typedef long long LL;
const int mod = 1e9 + 7;
int n;
int qmi(int a, int b, int c)
{
int res = 1;
while (b)
{
if (b & 1) res = (LL) res * a % mod;
a = (LL) a * a % mod;
b >>= 1;
}
return res;
}
int main()
{
scanf("%d", &n);
int x = 1, y = 1;
for (int i = 1; i <= 2 * n; i ++) x = (LL)x * i % mod;
for (int i = 1; i <= n; i ++) y = (LL)y * i % mod;
//要注意除(n + 1) 也要求逆元因为后面还要% mod
printf("%lld", (LL) x * qmi(y, mod - 2, mod) % mod * qmi(y, mod - 2, mod) % mod * qmi(n + 1, mod - 2, mod) % mod);
return 0;
}
本文来自互联网用户投稿,该文观点仅代表作者本人,不代表本站立场。本站仅提供信息存储空间服务,不拥有所有权,不承担相关法律责任。 如若内容造成侵权/违法违规/事实不符,请联系我的编程经验分享网邮箱:veading@qq.com进行投诉反馈,一经查实,立即删除!