代码随想录算法训练营DAY15|二叉树2

算法训练DAY15|二叉树2

层序遍历

学会二叉树的层序遍历，可以一口气打完以下十题：

• 102.二叉树的层序遍历

• 107.二叉树的层次遍历II

• 199.二叉树的右视图

• 637.二叉树的层平均值

• 429.N叉树的层序遍历

• 515.在每个树行中找最大值

• 116.填充每个节点的下一个右侧节点指针

• 117.填充每个节点的下一个右侧节点指针II

• 104.二叉树的最大深度

• 111.二叉树的最小深度

#102.二叉树的层序遍历

力扣题目链接

给你一个二叉树，请你返回其按 层序遍历 得到的节点值。 （即逐层地，从左到右访问所有节点）。

#思路

我们之前讲过了三篇关于二叉树的深度优先遍历的文章：

• 二叉树：前中后序递归法

• 二叉树：前中后序迭代法

• 二叉树：前中后序迭代方式统一写法

接下来我们再来介绍二叉树的另一种遍历方式：层序遍历。

层序遍历一个二叉树。就是从左到右一层一层的去遍历二叉树。这种遍历的方式和我们之前讲过的都不太一样。

需要借用一个辅助数据结构即队列来实现，队列先进先出，符合一层一层遍历的逻辑，而用栈先进后出适合模拟深度优先遍历也就是递归的逻辑。

而这种层序遍历方式就是图论中的广度优先遍历，只不过我们应用在二叉树上。

使用队列实现二叉树广度优先遍历，动画如下：

这样就实现了层序从左到右遍历二叉树。

代码如下：这份代码也可以作为二叉树层序遍历的模板，打十个就靠它了。

c++代码如下：

class Solution {
public:
 ? ?vector<vector<int>> levelOrder(TreeNode* root) {
 ? ? ? ?queue<TreeNode*> que;
 ? ? ? ?if (root != NULL) que.push(root);
 ? ? ? ?vector<vector<int>> result;
 ? ? ? ?while (!que.empty()) {
 ? ? ? ? ? ?int size = que.size();
 ? ? ? ? ? ?vector<int> vec;
 ? ? ? ? ? ?// 这里一定要使用固定大小size，不要使用que.size()，因为que.size是不断变化的
 ? ? ? ? ? ?for (int i = 0; i < size; i++) {
 ? ? ? ? ? ? ? ?TreeNode* node = que.front();
 ? ? ? ? ? ? ? ?que.pop();
 ? ? ? ? ? ? ? ?vec.push_back(node->val);
 ? ? ? ? ? ? ? ?if (node->left) que.push(node->left);
 ? ? ? ? ? ? ? ?if (node->right) que.push(node->right);
 ? ? ? ? ?  }
 ? ? ? ? ? ?result.push_back(vec);
 ? ? ?  }
 ? ? ? ?return result;
 ?  }
};

# 递归法
class Solution {
public:
 ? ?void order(TreeNode* cur, vector<vector<int>>& result, int depth)
 ?  {
 ? ? ? ?if (cur == nullptr) return;
 ? ? ? ?if (result.size() == depth) result.push_back(vector<int>());
 ? ? ? ?result[depth].push_back(cur->val);
 ? ? ? ?order(cur->left, result, depth + 1);
 ? ? ? ?order(cur->right, result, depth + 1);
 ?  }
 ? ?vector<vector<int>> levelOrder(TreeNode* root) {
 ? ? ? ?vector<vector<int>> result;
 ? ? ? ?int depth = 0;
 ? ? ? ?order(root, result, depth);
 ? ? ? ?return result;
 ?  }
};

199.二叉树的右视图

class Solution {
public:
 ? ?void order(TreeNode* root,vector<vector<int>> &vec,int depth){
 ? ? ? ?if(root==nullptr) return;
 ? ? ? ?if(depth == vec.size()) vec.push_back(vector<int>());
 ? ? ? ?vec[depth].push_back(root->val);
 ? ? ? ?order(root->left,vec,depth+1);
 ? ? ? ?order(root->right,vec,depth+1);
 ?  }
 ? ?vector<int> rightSideView(TreeNode* root) {
 ? ? ? ?vector<vector<int>> result;
 ? ? ? ?int depth = 0;
 ? ? ? ?order(root,result,depth);
 ? ? ? ?vector<int> result1;
 ? ? ? ?for(int i=0;i<result.size();i++){
 ? ? ? ? ? ?result1.push_back(result[i][result[i].size()-1]);
 ? ? ?  }
 ? ? ? ?return result1;
 ?  }
};
?
?
class Solution {
public:
 ? ?vector<int> rightSideView(TreeNode* root) {
 ? ? ? ?queue<TreeNode*> que; ? ? ?
 ? ? ? ?if(root!=nullptr) que.push(root);
 ? ? ? ?vector<int> vec;
 ? ? ? ?while(!que.empty()){
 ? ? ? ? ? ?int size = que.size(); ? ? ?    
 ? ? ? ? ? ?for(int i=0;i<size;i++){
 ? ? ? ? ? ? ? ?TreeNode* node = que.front();
 ? ? ? ? ?      que.pop();
 ? ? ? ? ? ? ? ?if(i==size-1){
 ? ? ? ? ? ? ? ? ? ?vec.push_back(node->val);
 ? ? ? ? ? ? ?  } ? ?
 ? ? ? ? ? ? ? ?if(node->left) que.push(node->left);
 ? ? ? ? ? ? ? ?if(node->right) que.push(node->right);
 ? ? ? ? ?  } ? ?
 ? ? ?  }
 ? ? ? ?return vec;
 ?  }
};

637.二叉树的层平均值

class Solution {
public:
 ? ?vector<double> averageOfLevels(TreeNode* root) {
 ? ? ? ?queue<TreeNode*> que;
 ? ? ? ?vector<double> result;
 ? ? ? ?if(root!=nullptr) que.push(root);
 ? ? ? ?while(!que.empty()){
 ? ? ? ? ? ?int size = que.size();
 ? ? ? ? ? ?long aver = 0;
 ? ? ? ? ? ?for(int i=0;i<size;i++){
 ? ? ? ? ? ? ? ?TreeNode* node = que.front();
 ? ? ? ? ? ? ? ?que.pop();
 ? ? ? ? ? ? ? ?aver+=node->val;
 ? ? ? ? ? ? ? ?if(node->left) que.push(node->left);
 ? ? ? ? ? ? ? ?if(node->right) que.push(node->right);
 ? ? ? ? ?  }
 ? ? ? ? ? ?result.push_back(aver*1.0/size);
 ? ? ?  }
 ? ? ? ?return result;
 ?  }
};

429.N叉树的层序遍历

力扣题目链接

给定一个 N 叉树，返回其节点值的层序遍历。 (即从左到右，逐层遍历)。

例如，给定一个 3叉树 :

返回其层序遍历:

[ [1], [3,2,4], [5,6] ]

#思路

这道题依旧是模板题，只不过一个节点有多个孩子了

C++代码:

class Solution {
public:
 ? ?vector<vector<int>> levelOrder(Node* root) {
 ? ? ? ?queue<Node*> que;
 ? ? ? ?if (root != NULL) que.push(root);
 ? ? ? ?vector<vector<int>> result;
 ? ? ? ?while (!que.empty()) {
 ? ? ? ? ? ?int size = que.size();
 ? ? ? ? ? ?vector<int> vec;
 ? ? ? ? ? ?for (int i = 0; i < size; i++) {
 ? ? ? ? ? ? ? ?Node* node = que.front();
 ? ? ? ? ? ? ? ?que.pop();
 ? ? ? ? ? ? ? ?vec.push_back(node->val);
 ? ? ? ? ? ? ? ?for (int i = 0; i < node->children.size(); i++) { // 将节点孩子加入队列
 ? ? ? ? ? ? ? ? ? ?if (node->children[i]) que.push(node->children[i]);
 ? ? ? ? ? ? ?  }
 ? ? ? ? ?  }
 ? ? ? ? ? ?result.push_back(vec);
 ? ? ?  }
 ? ? ? ?return result;
?
 ?  }
};

515.在每个树行中找最大值

力扣题目链接

您需要在二叉树的每一行中找到最大的值。

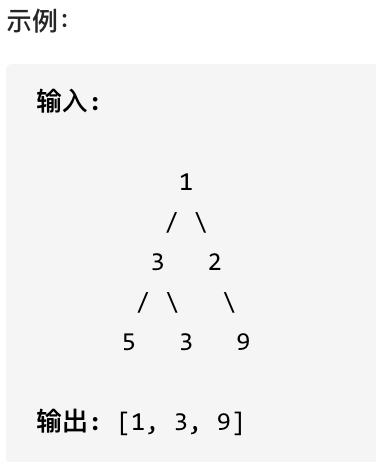
#思路

层序遍历，取每一层的最大值

C++代码：

class Solution {
public:
 ? ?vector<int> largestValues(TreeNode* root) {
 ? ? ? ?queue<TreeNode*> que;
 ? ? ? ?if (root != NULL) que.push(root);
 ? ? ? ?vector<int> result;
 ? ? ? ?while (!que.empty()) {
 ? ? ? ? ? ?int size = que.size();
 ? ? ? ? ? ?int maxValue = INT_MIN; // 取每一层的最大值
 ? ? ? ? ? ?for (int i = 0; i < size; i++) {
 ? ? ? ? ? ? ? ?TreeNode* node = que.front();
 ? ? ? ? ? ? ? ?que.pop();
 ? ? ? ? ? ? ? ?maxValue = node->val > maxValue ? node->val : maxValue;
 ? ? ? ? ? ? ? ?if (node->left) que.push(node->left);
 ? ? ? ? ? ? ? ?if (node->right) que.push(node->right);
 ? ? ? ? ?  }
 ? ? ? ? ? ?result.push_back(maxValue); // 把最大值放进数组
 ? ? ?  }
 ? ? ? ?return result;
 ?  }
};

116.填充每个节点的下一个右侧节点指针

力扣题目链接(opens new window)

给定一个完美二叉树，其所有叶子节点都在同一层，每个父节点都有两个子节点。二叉树定义如下：

struct Node {
 ?int val;
 ?Node *left;
 ?Node *right;
 ?Node *next;
}

1 2 3 4 5 6

填充它的每个 next 指针，让这个指针指向其下一个右侧节点。如果找不到下一个右侧节点，则将 next 指针设置为 NULL。

初始状态下，所有 next 指针都被设置为 NULL。

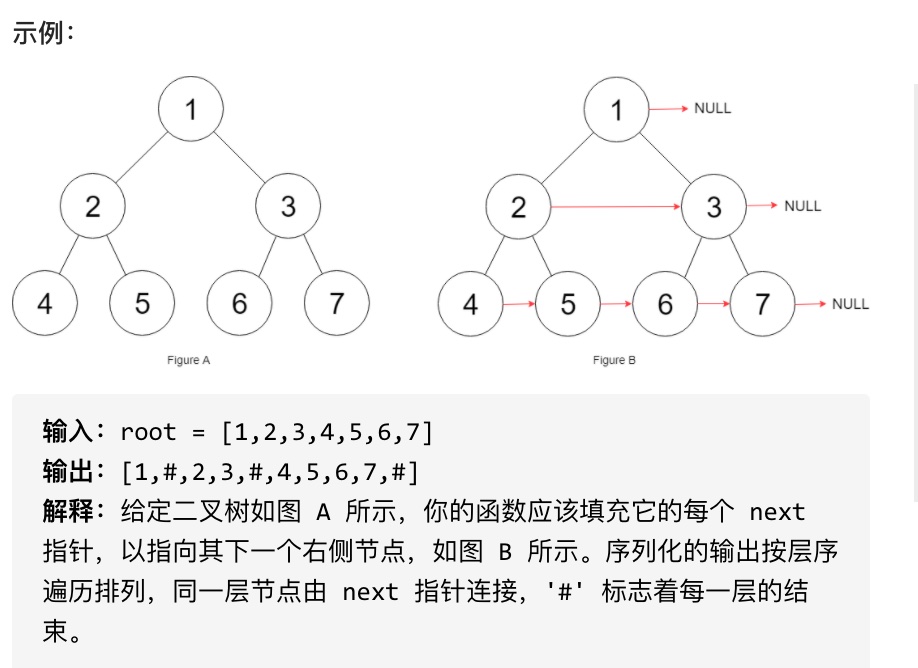
#思路

本题依然是层序遍历，只不过在单层遍历的时候记录一下本层的头部节点，然后在遍历的时候让前一个节点指向本节点就可以了

C++代码：

class Solution {
public:
 ? ?Node* connect(Node* root) {
 ? ? ? ?queue<Node*> que;
 ? ? ? ?if (root != NULL) que.push(root);
 ? ? ? ?while (!que.empty()) {
 ? ? ? ? ? ?int size = que.size();
 ? ? ? ? ? ?// vector<int> vec;
 ? ? ? ? ? ?Node* nodePre;
 ? ? ? ? ? ?Node* node;
 ? ? ? ? ? ?for (int i = 0; i < size; i++) {
 ? ? ? ? ? ? ? ?if (i == 0) {
 ? ? ? ? ? ? ? ? ? ?nodePre = que.front(); // 取出一层的头结点
 ? ? ? ? ? ? ? ? ? ?que.pop();
 ? ? ? ? ? ? ? ? ? ?node = nodePre;
 ? ? ? ? ? ? ?  } else {
 ? ? ? ? ? ? ? ? ? ?node = que.front();
 ? ? ? ? ? ? ? ? ? ?que.pop();
 ? ? ? ? ? ? ? ? ? ?nodePre->next = node; // 本层前一个节点next指向本节点
 ? ? ? ? ? ? ? ? ? ?nodePre = nodePre->next;
 ? ? ? ? ? ? ?  }
 ? ? ? ? ? ? ? ?if (node->left) que.push(node->left);
 ? ? ? ? ? ? ? ?if (node->right) que.push(node->right);
 ? ? ? ? ?  }
 ? ? ? ? ? ?nodePre->next = NULL; // 本层最后一个节点指向NULL
 ? ? ?  }
 ? ? ? ?return root;
?
 ?  }
};

117.填充每个节点的下一个右侧节点指针II

力扣题目链接

#思路

这道题目说是二叉树，但116题目说是完整二叉树，其实没有任何差别，一样的代码一样的逻辑一样的味道

C++代码：

class Solution {
public:
 ? ?Node* connect(Node* root) {
 ? ? ? ?queue<Node*> que;
 ? ? ? ?if (root != NULL) que.push(root);
 ? ? ? ?while (!que.empty()) {
 ? ? ? ? ? ?int size = que.size();
 ? ? ? ? ? ?vector<int> vec;
 ? ? ? ? ? ?Node* nodePre;
 ? ? ? ? ? ?Node* node;
 ? ? ? ? ? ?for (int i = 0; i < size; i++) {
 ? ? ? ? ? ? ? ?if (i == 0) {
 ? ? ? ? ? ? ? ? ? ?nodePre = que.front(); // 取出一层的头结点
 ? ? ? ? ? ? ? ? ? ?que.pop();
 ? ? ? ? ? ? ? ? ? ?node = nodePre;
 ? ? ? ? ? ? ?  } else {
 ? ? ? ? ? ? ? ? ? ?node = que.front();
 ? ? ? ? ? ? ? ? ? ?que.pop();
 ? ? ? ? ? ? ? ? ? ?nodePre->next = node; // 本层前一个节点next指向本节点
 ? ? ? ? ? ? ? ? ? ?nodePre = nodePre->next;
 ? ? ? ? ? ? ?  }
 ? ? ? ? ? ? ? ?if (node->left) que.push(node->left);
 ? ? ? ? ? ? ? ?if (node->right) que.push(node->right);
 ? ? ? ? ?  }
 ? ? ? ? ? ?nodePre->next = NULL; // 本层最后一个节点指向NULL
 ? ? ?  }
 ? ? ? ?return root;
 ?  }
};

104.二叉树的最大深度

力扣题目链接

给定一个二叉树，找出其最大深度。

二叉树的深度为根节点到最远叶子节点的最长路径上的节点数。

说明: 叶子节点是指没有子节点的节点。

示例：

给定二叉树 [3,9,20,null,null,15,7]，

返回它的最大深度 3 。

#思路

使用迭代法的话，使用层序遍历是最为合适的，因为最大的深度就是二叉树的层数，和层序遍历的方式极其吻合。

在二叉树中，一层一层的来遍历二叉树，记录一下遍历的层数就是二叉树的深度，如图所示：

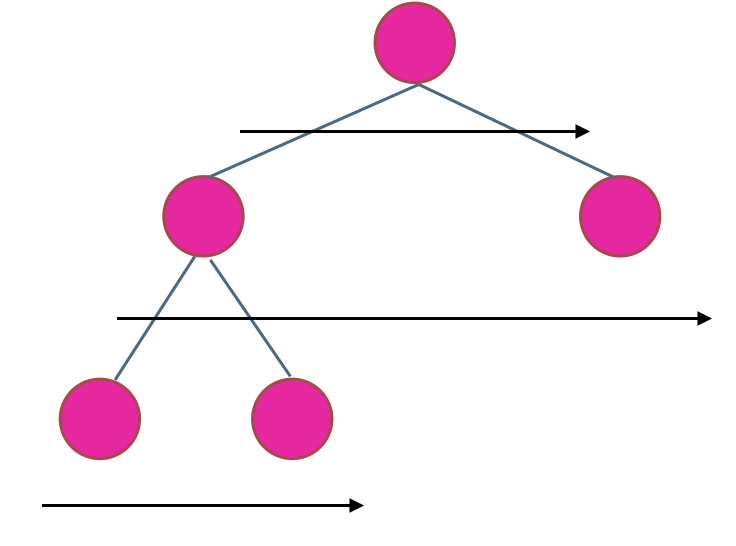
所以这道题的迭代法就是一道模板题，可以使用二叉树层序遍历的模板来解决的。

C++代码如下：

class Solution {
public:
 ? ?int maxDepth(TreeNode* root) {
 ? ? ? ?if (root == NULL) return 0;
 ? ? ? ?int depth = 0;
 ? ? ? ?queue<TreeNode*> que;
 ? ? ? ?que.push(root);
 ? ? ? ?while(!que.empty()) {
 ? ? ? ? ? ?int size = que.size();
 ? ? ? ? ? ?depth++; // 记录深度
 ? ? ? ? ? ?for (int i = 0; i < size; i++) {
 ? ? ? ? ? ? ? ?TreeNode* node = que.front();
 ? ? ? ? ? ? ? ?que.pop();
 ? ? ? ? ? ? ? ?if (node->left) que.push(node->left);
 ? ? ? ? ? ? ? ?if (node->right) que.push(node->right);
 ? ? ? ? ?  }
 ? ? ?  }
 ? ? ? ?return depth;
 ?  }
};

111.二叉树的最小深度

力扣题目链接

#思路

相对于 104.二叉树的最大深度 ，本题还也可以使用层序遍历的方式来解决，思路是一样的。

需要注意的是，只有当左右孩子都为空的时候，才说明遍历的最低点了。如果其中一个孩子为空则不是最低点

代码如下：（详细注释）

class Solution {
public:
 ? ?int minDepth(TreeNode* root) {
 ? ? ? ?if (root == NULL) return 0;
 ? ? ? ?int depth = 0;
 ? ? ? ?queue<TreeNode*> que;
 ? ? ? ?que.push(root);
 ? ? ? ?while(!que.empty()) {
 ? ? ? ? ? ?int size = que.size();
 ? ? ? ? ? ?depth++; // 记录最小深度
 ? ? ? ? ? ?for (int i = 0; i < size; i++) {
 ? ? ? ? ? ? ? ?TreeNode* node = que.front();
 ? ? ? ? ? ? ? ?que.pop();
 ? ? ? ? ? ? ? ?if (node->left) que.push(node->left);
 ? ? ? ? ? ? ? ?if (node->right) que.push(node->right);
 ? ? ? ? ? ? ? ?if (!node->left && !node->right) { // 当左右孩子都为空的时候，说明是最低点的一层了，退出
 ? ? ? ? ? ? ? ? ? ?return depth;
 ? ? ? ? ? ? ?  }
 ? ? ? ? ?  }
 ? ? ?  }
 ? ? ? ?return depth;
 ?  }
};

226.翻转二叉树

力扣题目链接

翻转一棵二叉树。

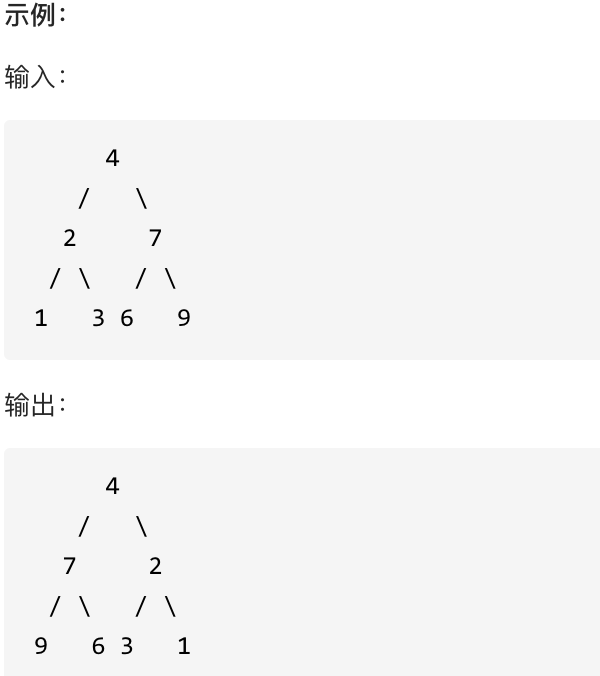
广度优先遍历

也就是层序遍历，层数遍历也是可以翻转这棵树的，因为层序遍历也可以把每个节点的左右孩子都翻转一遍，代码如下：

class Solution {
public:
 ? ?TreeNode* invertTree(TreeNode* root) {
 ? ? ? ?queue<TreeNode*> que;
 ? ? ? ?if (root != NULL) que.push(root);
 ? ? ? ?while (!que.empty()) {
 ? ? ? ? ? ?int size = que.size();
 ? ? ? ? ? ?for (int i = 0; i < size; i++) {
 ? ? ? ? ? ? ? ?TreeNode* node = que.front();
 ? ? ? ? ? ? ? ?que.pop();
 ? ? ? ? ? ? ? ?swap(node->left, node->right); // 节点处理
 ? ? ? ? ? ? ? ?if (node->left) que.push(node->left);
 ? ? ? ? ? ? ? ?if (node->right) que.push(node->right);
 ? ? ? ? ?  }
 ? ? ?  }
 ? ? ? ?return root;
 ?  }
};

我们来看一下递归三部曲：

• 确定递归函数的参数和返回值

参数就是要传入节点的指针，不需要其他参数了，通常此时定下来主要参数，如果在写递归的逻辑中发现还需要其他参数的时候，随时补充。

返回值的话其实也不需要，但是题目中给出的要返回root节点的指针，可以直接使用题目定义好的函数，所以就函数的返回类型为TreeNode*。

TreeNode* invertTree(TreeNode* root)

• 确定终止条件

当前节点为空的时候，就返回

if (root == NULL) return root;

• 确定单层递归的逻辑

因为是先前序遍历，所以先进行交换左右孩子节点，然后反转左子树，反转右子树。

swap(root->left, root->right);
invertTree(root->left);
invertTree(root->right);

基于这递归三步法，代码基本写完，C++代码如下：

class Solution {
public:
 ? ?TreeNode* invertTree(TreeNode* root) {
 ? ? ? ?if (root == NULL) return root;
 ? ? ? ?swap(root->left, root->right); ?// 中
 ? ? ? ?invertTree(root->left); ? ? ? ? // 左
 ? ? ? ?invertTree(root->right); ? ? ? ?// 右
 ? ? ? ?return root;
 ?  }
};

#迭代法

跳过

101. 对称二叉树

力扣题目链接

给定一个二叉树，检查它是否是镜像对称的。

最后递归的C++整体代码如下：

class Solution {
public:
 ? ?bool compare(TreeNode* left, TreeNode* right) {
 ? ? ? ?// 首先排除空节点的情况
 ? ? ? ?if (left == NULL && right != NULL) return false;
 ? ? ? ?else if (left != NULL && right == NULL) return false;
 ? ? ? ?else if (left == NULL && right == NULL) return true;
 ? ? ? ?// 排除了空节点，再排除数值不相同的情况
 ? ? ? ?else if (left->val != right->val) return false;
?
 ? ? ? ?// 此时就是：左右节点都不为空，且数值相同的情况
 ? ? ? ?// 此时才做递归，做下一层的判断
 ? ? ? ?bool outside = compare(left->left, right->right); ? // 左子树：左、 右子树：右
 ? ? ? ?bool inside = compare(left->right, right->left); ? ?// 左子树：右、 右子树：左
 ? ? ? ?bool isSame = outside && inside; ? ? ? ? ? ? ? ? ? ?// 左子树：中、 右子树：中 （逻辑处理）
 ? ? ? ?return isSame;
?
 ?  }
 ? ?bool isSymmetric(TreeNode* root) {
 ? ? ? ?if (root == NULL) return true;
 ? ? ? ?return compare(root->left, root->right);
 ?  }
};

迭代法

跳过