刷题记录Day18-二叉树(找树左下角的值、路径总和、从中序与后序遍历序列构造二叉树)
刷题记录Day18-二叉树(找树左下角的值、路径总和、从中序与后序遍历序列构造二叉树)
文章目录
前言
题目来源:leetcode
刷题顺序:代码随想录
刷题工具:VSCode+leetcode插件
补充:延毕时间充裕,会结合LeetCode 101: A LeetCode Grinding Guide (C++ Version)相似题目一起做。
一、找树左下角的值
1. 513找树左下角的值
题目:
给定一个二叉树的 根节点 root,请找出该二叉树的 最底层 最左边 节点的值。
假设二叉树中至少有一个节点。
示例:
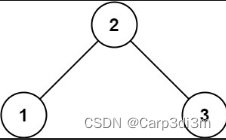
输入: root = [2,1,3]
输出: 1
代码:
方法一,前序遍历递归回溯
class Solution {
public:
int maxDepth = INT_MIN;
int res;
void getLeftNum (TreeNode* cur, int depth){
int left;
if (cur->left == NULL && cur->right == NULL){ //中
if (depth > maxDepth){
maxDepth = depth;
res = cur->val;
return;
}
}
if (cur->left){ //左
++depth;
getLeftNum(cur->left, depth);
--depth; //回溯
}
if (cur->right){ // 右
++depth;
getLeftNum(cur->right, depth);
--depth; //回溯
}
return;
}
int findBottomLeftValue(TreeNode* root) {
getLeftNum(root, 0);
return res;
}
};
方法二,层序遍历,记录最后一行第一个值
class Solution {
public:
int findBottomLeftValue(TreeNode* root) {
int res;
queue<TreeNode*> que;
if (root != NULL) que.push(root);
while (!que.empty()){
int size = que.size();
for (int i = 0; i< size; ++i){
TreeNode* node = que.front();
que.pop();
if (i == 0) res = node->val; //记录最后一行第一个值
if (node->left) que.push(node->left);
if (node->right) que.push(node->right);
}
}
return res;
}
};
二、路径总和
1. 112路经总和
题目:
给你二叉树的根节点 root 和一个表示目标和的整数 targetSum 。判断该树中是否存在 根节点到叶子节点 的路径,这条路径上所有节点值相加等于目标和 targetSum 。如果存在,返回 true ;否则,返回 false 。
叶子节点 是指没有子节点的节点。
示例:

输入:root = [5,4,8,11,null,13,4,7,2,null,null,null,1], targetSum = 22
输出:true
解释:等于目标和的根节点到叶节点路径如上图所示。
代码:
方法一,自己写的前序递归回溯
class Solution {
public:
void getPathSum(TreeNode* cur, int& sum, vector<int>& pathSum){
sum += cur->val; //中
if (cur->left == NULL && cur->right == NULL){ //中止条件
pathSum.push_back(sum);
return;
}
if (cur->left){ //左
getPathSum(cur->left, sum, pathSum);
sum -= cur->left->val;
}
if (cur->right){ //右
getPathSum(cur->right, sum, pathSum);
sum -= cur->right->val;
}
return;
}
bool hasPathSum(TreeNode* root, int targetSum) {
int sum = 0;
vector<int> pathSum;
if (root == NULL) return false;
getPathSum(root, sum, pathSum);
for (auto i : pathSum){
if (i == targetSum) return true;
}
return false;
}
};
方法二,代码随想录上的方法
这个方法确实更快,不需要遍历找所有的路径和,用目标值减去当前节点,当目标值变成0且节点已经是叶子节点时,直接返回
class Solution {
private:
bool traversal(TreeNode* cur, int count) {
if (!cur->left && !cur->right && count == 0) return true; // 遇到叶子节点,并且计数为0
if (!cur->left && !cur->right) return false; // 遇到叶子节点直接返回
if (cur->left) { // 左
count -= cur->left->val; // 递归,处理节点;
if (traversal(cur->left, count)) return true;
count += cur->left->val; // 回溯,撤销处理结果
}
if (cur->right) { // 右
count -= cur->right->val; // 递归,处理节点;
if (traversal(cur->right, count)) return true;
count += cur->right->val; // 回溯,撤销处理结果
}
return false;
}
public:
bool hasPathSum(TreeNode* root, int sum) {
if (root == NULL) return false;
return traversal(root, sum - root->val);
}
};
2. 113路径总和II
题目:
给你二叉树的根节点 root 和一个整数目标和 targetSum ,找出所有 从根节点到叶子节点 路径总和等于给定目标和的路径。
叶子节点 是指没有子节点的节点。
示例:

输入:root = [5,4,8,11,null,13,4,7,2,null,null,5,1], targetSum = 22
输出:[[5,4,11,2],[5,8,4,5]]
代码:
方法一,自己写的前序递归回溯
lass Solution {
public:
void getPathSum(TreeNode* cur, vector<int>& path, vector<vector<int>>& pathAll){
path.push_back(cur->val);
if (cur->left == NULL && cur->right == NULL){ //中止条件
pathAll.push_back(path);
return;
}
if (cur->left){ //左
getPathSum(cur->left, path, pathAll);
path.pop_back();
}
if (cur->right){ //右
getPathSum(cur->right, path, pathAll);
path.pop_back();
}
return;
}
vector<vector<int>> pathSum(TreeNode* root, int targetSum) {
vector<int> path;
vector<vector<int>> pathAll;
vector<vector<int>> res;
if (root == NULL) return pathAll;
getPathSum(root, path, pathAll);
for (auto i : pathAll){
if (accumulate(i.begin(),i.end(),0) == targetSum){
res.push_back(i);
}
}
return res;
}
};
方法二,代码随想录的方法
class Solution {
public:
vector<int> path;
vector<vector<int>> res;
void traversal(TreeNode* cur, int count){
if (cur->left == NULL && cur->right == NULL && count == 0){ //中止条件
res.push_back(path);
return;
}
if (cur->left){ //左
count -= cur->left->val;
path.push_back(cur->left->val);
traversal(cur->left, count);
count += cur->left->val;
path.pop_back();
}
if (cur->right){ //右
count -= cur->right->val;
path.push_back(cur->right->val);
traversal(cur->right, count);
count += cur->right->val;
path.pop_back();
}
return;
}
vector<vector<int>> pathSum(TreeNode* root, int targetSum) {
if (root == NULL) return res;
path.push_back(root->val);
traversal(root, targetSum - root->val);
return res;
}
};
三、 从中序与后序遍历序列构造二叉树
1. 106从中序与后序遍历序列构造二叉树
题目:
给定两个整数数组 inorder 和 postorder ,其中 inorder 是二叉树的中序遍历, postorder 是同一棵树的后序遍历,请你构造并返回这颗 二叉树 。
示例:
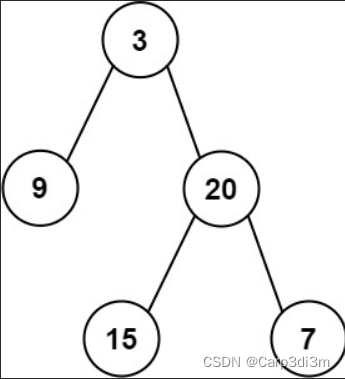
输入:inorder = [9,3,15,20,7], postorder = [9,15,7,20,3]
输出:[3,9,20,null,null,15,7]
代码:
class Solution {
public:
TreeNode* traversal(vector<int>& inorder, vector<int>& postorder){
if (postorder.size() == 0) return NULL;
//第一步,我先在后序数组中的最后一位找到根节点(中)
int rootval = postorder[postorder.size()-1];
TreeNode* root = new TreeNode(rootval);
if (postorder.size() == 1) return root;
//第二步骤,根据节点值找到它在中序数组的位置index
int index;
for (index = 0; index < inorder.size(); ++index){
if (inorder[index] == rootval) break;
}
//第三步,根据index切割中序数组--->得到左中序、右中序
vector<int> leftInorder(inorder.begin(), inorder.begin() + index);
vector<int> rightInorder(inorder.begin() + index + 1, inorder.end());
//第四步,根据左中序大小切割后序数组
postorder.resize(postorder.size()-1);
vector<int> leftPostorder(postorder.begin(), postorder.begin() + leftInorder.size());
vector<int> rightPostorder(postorder.begin() + leftInorder.size(), postorder.end());
//迭代
root->left = traversal(leftInorder, leftPostorder);
root->right = traversal(rightInorder, rightPostorder);
return root;
}
TreeNode* buildTree(vector<int>& inorder, vector<int>& postorder) {
if (inorder.size() == 0 || postorder.size() == 0) return NULL;
return traversal(inorder, postorder);
}
};
2. 105从前序与中序遍历序列构造二叉树
题目:
给定两个整数数组 preorder 和 inorder ,其中 preorder 是二叉树的先序遍历, inorder 是同一棵树的中序遍历,请构造二叉树并返回其根节点。
示例:

输入: preorder = [3,9,20,15,7], inorder = [9,3,15,20,7]
输出: [3,9,20,null,null,15,7]
代码:
class Solution {
public:
TreeNode* traversal(vector<int>& preorder, vector<int>& inorder){
if (preorder.size() == 0) return NULL;
//第一步,找到根节点
int rootval = preorder[0];
TreeNode* root = new TreeNode(rootval);
if (preorder.size() == 1) return root;
//第二步,根据节点值找到中序遍历的index
int index;
for (index = 0; index < inorder.size(); ++index){
if (inorder[index] == rootval) break;
}
//第三步,根据index切割中序数组--->得到左中序、右中序
vector<int> leftInorder(inorder.begin(), inorder.begin() + index);
vector<int> rightInorder(inorder.begin() + index +1, inorder.end());
//第四步,根据左中序切割前序
preorder.erase(preorder.begin());
vector<int> leftPreorder(preorder.begin(), preorder.begin() + leftInorder.size());
vector<int> rightPreorder(preorder.begin() + leftInorder.size(), preorder.end());
//递归
root->left = traversal(leftPreorder, leftInorder);
root->right = traversal(rightPreorder, rightInorder);
return root;
}
TreeNode* buildTree(vector<int>& preorder, vector<int>& inorder) {
if (inorder.size() == 0 || preorder.size() == 0) return NULL;
return traversal(preorder, inorder);
}
};
总结
今天递归好像稍微好一点了。
本文来自互联网用户投稿,该文观点仅代表作者本人,不代表本站立场。本站仅提供信息存储空间服务,不拥有所有权,不承担相关法律责任。 如若内容造成侵权/违法违规/事实不符,请联系我的编程经验分享网邮箱:veading@qq.com进行投诉反馈,一经查实,立即删除!