力扣日记12.13-【二叉树篇】从中序与后序遍历序列构造二叉树

力扣日记：【二叉树篇】从中序与后序遍历序列构造二叉树

日期：2023.12.13
参考：代码随想录、力扣

106. 从中序与后序遍历序列构造二叉树

题目描述

难度：中等

给定两个整数数组 inorder 和 postorder ，其中 inorder 是二叉树的中序遍历， postorder 是同一棵树的后序遍历，请你构造并返回这颗 二叉树 。

示例 1:

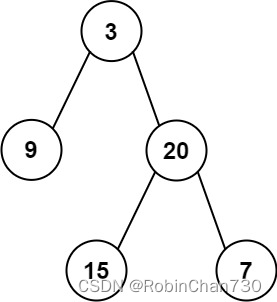
输入：inorder = [9,3,15,20,7], postorder = [9,15,7,20,3]
输出：[3,9,20,null,null,15,7]

示例 2:

输入：inorder = [-1], postorder = [-1]
输出：[-1]

提示:

• 1 <= inorder.length <= 3000
• postorder.length == inorder.length
• -3000 <= inorder[i], postorder[i] <= 3000
• inorder 和 postorder 都由 不同 的值组成
• postorder 中每一个值都在 inorder 中
• inorder 保证是树的中序遍历
• postorder 保证是树的后序遍历

题解

/**
 * Definition for a binary tree node.
 * struct TreeNode {
 *     int val;
 *     TreeNode *left;
 *     TreeNode *right;
 *     TreeNode() : val(0), left(nullptr), right(nullptr) {}
 *     TreeNode(int x) : val(x), left(nullptr), right(nullptr) {}
 *     TreeNode(int x, TreeNode *left, TreeNode *right) : val(x), left(left), right(right) {}
 * };
 */
class Solution {
# define SOLUTION 2
public:
# if SOLUTION == 1
    TreeNode* buildTree(vector<int>& inorder, vector<int>& postorder) {
        // 递归返回值与参数：返回值为中节点, 参数为中序和后序数组
        // 从中序与后序遍历序列构造二叉树的步骤
        // 1. 如果后序数组为空, 则为空节点(终止条件)
        if (postorder.size() == 0) return nullptr;  // 为空节点
        // 2. 根据后序数组最后一个值得到中节点值
        int nodeVal = postorder[postorder.size() - 1];  // 后序数组最后一个值为中节点值
        TreeNode* node = new TreeNode(nodeVal); // 构造中节点
        // 注意如果是叶子节点, 则不需要再去切割, 直接返回当前节点
        if (postorder.size() == 1) return node;
        // 3. 寻找中序数组位置作切割点
        int index = 0;
        for (int i = 0; i < inorder.size(); i++) {
            if (inorder[i] == nodeVal) {
                index = i;  
                // index 为中节点值对应序号, 则[0, index)为左子树, [index + 1, size)为右子树, 注意统一区间开闭 
            }
        }
        // 4. 根据此切割点对中序数组进行切割
        vector<int> inorderLeft(inorder.begin(), inorder.begin() + index);  // [0, index)
        vector<int> inorderRight(inorder.begin() + index + 1, inorder.end());   // [index + 1, size)
        // 5. 再根据切割后中序数组左右区间长度对后序数组进行切割
        vector<int> postorderLeft(postorder.begin(), postorder.begin() + inorderLeft.size()); // [0, left.size)
        vector<int> postorderRight(postorder.begin() + inorderLeft.size(), postorder.begin() + inorderLeft.size() + inorderRight.size()); // [left.size, left.size + right.size)
        // 6. 将中序数组与后序数组的左子树区间进行递归处理, 递归返回值为左子树的根节点,作为当前node左节点
        node->left = buildTree(inorderLeft, postorderLeft);   // 中序和后序的左子树遍历数组都分别按中序和后序遍历
        // 7. 将中序数组与后序数组的右子树区间进行递归处理
        node->right = buildTree(inorderRight, postorderRight);
        return node;    // 返回已经接上左右节点的中节点
    }
# elif SOLUTION == 2    // 优化：用下标索引，不需要每次构建子数组
    TreeNode* buildTree(vector<int>& inorder, vector<int>& postorder) {
        int inorderBegin = 0, inorderEnd = inorder.size();  // [0, size)
        int postorderBegin = 0, postorderEnd = postorder.size();    // [0, size)
        return traversal(inorder, inorderBegin, inorderEnd, postorder, postorderBegin, postorderEnd);
    }
    TreeNode* traversal (vector<int>& inorder, int inorderBegin, int inorderEnd, vector<int>& postorder, int postorderBegin, int postorderEnd) {
        // 递归返回值与参数：返回值为中节点, 参数为原始中序后序数组, 以及用来表示当前中后序数组的下标
        // 不变量：左闭右开
        // 从中序与后序遍历序列构造二叉树的步骤
        // 1. 如果当前后序数组为空, 则为空节点(终止条件)
        if (postorderEnd - postorderBegin == 0) return nullptr;  // 为空节点 [Begin, Begin)
        // 2. 根据后序数组最后一个值得到中节点值
        int nodeVal = postorder[postorderEnd - 1];  // 后序数组最后一个值为中节点值, 右开, 则需-1
        TreeNode* node = new TreeNode(nodeVal); // 构造中节点
        // 注意如果是叶子节点, 则不需要再去切割, 直接返回当前节点
        if (postorderEnd - postorderBegin == 1) return node;
        // 3. 寻找中序数组位置作切割点
        int index = 0;
        for (int i = inorderBegin; i < inorderEnd; i++) {
            if (inorder[i] == nodeVal) {
                index = i;  
                // index 为中节点值对应序号, 则[inorderBegin, index)为左子树, [index + 1, inorderEnd)为右子树, 注意统一区间开闭 
            }
        }
        // 4. 根据此切割点对中序数组进行切割
        int inorderLeftBegin = inorderBegin; // 左子树区间的开始下标(左闭)
        int inorderLeftEnd = index; // 左子树区间的结束下标(右开)
        int inorderRightBegin = index + 1;  // 右子树区间
        int inorderRightEnd = inorderEnd;
        // 5. 再根据切割后中序数组左右区间长度对后序数组进行切割
        int LeftSize = inorderLeftEnd - inorderLeftBegin;    // 左子树大小
        int postorderLeftBegin = postorderBegin;
        int postorderLeftEnd = postorderBegin + LeftSize;    // 后序与中序的左子树数组大小一致, LeftSize: [postorderLeftBegin, postorderLeftBegin + LeftSize)
        int RightSize = inorderRightEnd - inorderRightBegin;
        int postorderRightBegin = postorderLeftEnd; // 左闭
        int postorderRightEnd = postorderLeftEnd + RightSize;
        // 6. 将中序数组与后序数组的左子树区间进行递归处理, 递归返回值为左子树的根节点,作为当前node左节点
        node->left = traversal(inorder, inorderLeftBegin, inorderLeftEnd, postorder, postorderLeftBegin, postorderLeftEnd);   // 中序和后序的左子树遍历数组都分别按中序和后序遍历
        // 7. 将中序数组与后序数组的右子树区间进行递归处理
        node->right = traversal(inorder, inorderRightBegin, inorderRightEnd, postorder, postorderRightBegin, postorderRightEnd);
        return node;    // 返回已经接上左右节点的中节点
    }
# endif
};

复杂度

时间复杂度：
空间复杂度：

思路总结

• 从中序与后序遍历序列构造二叉树的步骤
  • 1. 如果后序数组为空, 则为空节点
    2. 根据后序数组最后一个值得到中节点值
    3. 寻找中序数组位置作切割点
    4. 根据此切割点对中序数组进行切割
    5. 再根据切割后中序数组左右区间长度对后序数组进行切割
    6. 将中序数组与后序数组的左子树区间进行递归处理(两个左子树区间数组分别为左子树的中序数组和后序数组)
    7. 将中序数组与后序数组的右子树区间进行递归处理
• 示意图
  
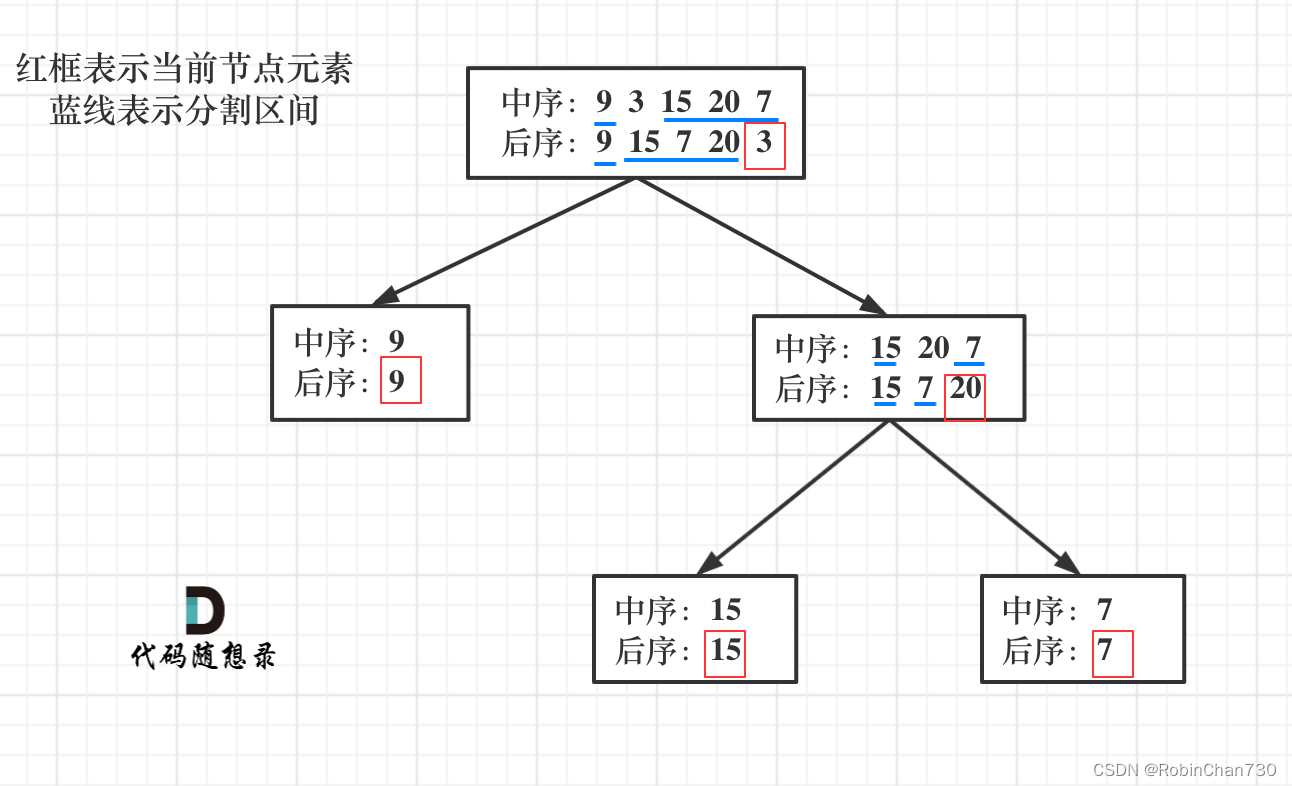
• 对于第二种解法，即用下标索引表示子数组（而不是直接构造子数组），要分别确定好左右子树的中序和后序数组的开始、结束下标。统一用左闭右开来表示。相对繁琐一些，但时间和空间复杂度更优化。

相关题目：105. 从前序与中序遍历序列构造二叉树

题目描述

难度：中等

给定两个整数数组 preorder 和 inorder ，其中 preorder 是二叉树的先序遍历， inorder 是同一棵树的中序遍历，请构造二叉树并返回其根节点。

示例 1:

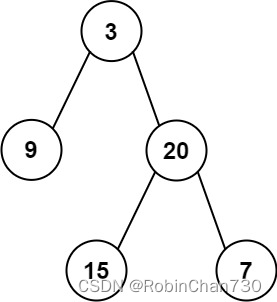
输入: preorder = [3,9,20,15,7], inorder = [9,3,15,20,7]
输出: [3,9,20,null,null,15,7]

示例 2:

输入: preorder = [-1], inorder = [-1]
输出: [-1]

提示:

• 1 <= preorder.length <= 3000
• inorder.length == preorder.length
• -3000 <= preorder[i], inorder[i] <= 3000
• preorder 和 inorder 均 无重复 元素
• inorder 均出现在 preorder
• preorder 保证 为二叉树的前序遍历序列
• inorder 保证 为二叉树的中序遍历序列

题解

/**
 * Definition for a binary tree node.
 * struct TreeNode {
 *     int val;
 *     TreeNode *left;
 *     TreeNode *right;
 *     TreeNode() : val(0), left(nullptr), right(nullptr) {}
 *     TreeNode(int x) : val(x), left(nullptr), right(nullptr) {}
 *     TreeNode(int x, TreeNode *left, TreeNode *right) : val(x), left(left), right(right) {}
 * };
 */
class Solution {
public:
    TreeNode* buildTree(vector<int>& preorder, vector<int>& inorder) {
        // 1. 如果前序数组为空, 则为空节点
        if (preorder.size() == 0)   return nullptr;
        // 2. 根据前序数组第一个值得到中节点值
        int nodeVal = preorder[0];
        // 构造中节点
        TreeNode* node = new TreeNode(nodeVal);
        // 如果是叶子节点直接返回
        if (preorder.size() == 1)   return node;
        // 3. 寻找中序数组位置作切割点
        int index = 0;
        for (int i = 0; i < inorder.size(); i++) {
            if (inorder[i] == nodeVal) {
                index = i;
            }
        }
        // 4. 根据此切割点对中序数组进行切割
        vector<int> inorderLeft(inorder.begin(), inorder.begin() + index);
        vector<int> inorderRight(inorder.begin() + index + 1, inorder.end());
        // 5. 再根据切割后的中序数组左右区间长度对前序数组进行切割
        vector<int> preorderLeft(preorder.begin() + 1, preorder.begin() + 1 + inorderLeft.size());  // 第二个值开始
        vector<int> preorderRight(preorder.begin() + 1 + inorderLeft.size(), preorder.end());
        // 6. 将中序数组与前序数组的左子树区间进行递归处理(两个左子树区间数组分别为左子树的中序数组和前序数组)
        node->left = buildTree(preorderLeft, inorderLeft);
        // 7. 将中序数组与前序数组的右子树区间进行递归处理
        node->right = buildTree(preorderRight, inorderRight);
        return node;
    }
};

复杂度

思路总结

• 与 从后序与中序遍历序列构造二叉树 的思路基本一致
• 从前序与中序遍历序列构造二叉树的步骤（前序：中左右；中序：左中右）
  • 1. 如果前序数组为空, 则为空节点
    2. 根据前序数组第一个值得到中节点值
    3. 寻找中序数组位置作切割点
    4. 根据此切割点对中序数组进行切割
    5. 再根据切割后的中序数组左右区间长度对前序数组进行切割
    6. 将中序数组与前序数组的左子树区间进行递归处理(两个左子树区间数组分别为左子树的中序数组和前序数组)
    7. 将中序数组与前序数组的右子树区间进行递归处理
• 这里要明确一点：前序和中序、后序和中序都可以分别唯一确定一棵二叉树；但前序和后序不能唯一确定一棵二叉树！因为没有中序遍历无法确定左右部分，也就是无法分割。
  • 如
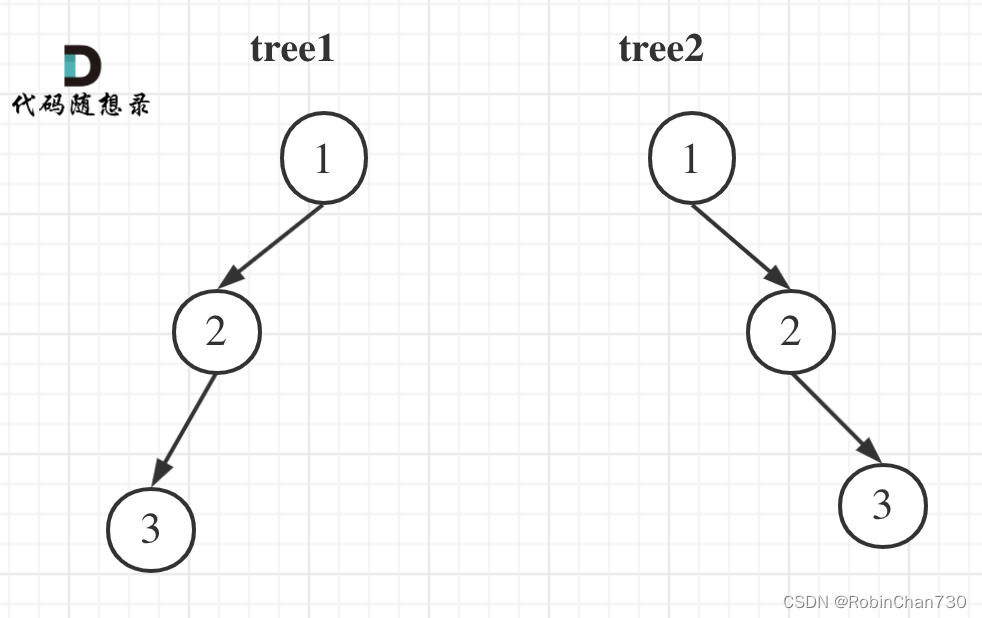
  • 上面两棵树的前序、后序都分别相等